Interior Angles Of Polygons Math Is Fun
Mar 16, 2016 learn how to find the interior and exterior angles of a polygon in this geometry interior and exterior angles free math we discuss regular and nonregular polygons and go over the . The interior angle concept can be extended in a and concave polygons k = 1, since the exterior angle sum is . In geometry, you can find the sum of the interior or exterior angles of a polygon based on the number of sides the polygon has. you can then apply this information to find individual interior or exterior angles. the sum of the exterior angles of any polygon is 360 degrees. the formula tells you the
Review Interior And Exterior Angles Of Polygons Geometry Ixl
Theorems include: measures of interior angles of a triangle sum to 180°; base angles of isosceles triangles are congruent; the segment joining midpoints of two sides of a triangle is parallel to the third side and half the length; the medians of a triangle meet at a point. worksheets: regents-interior and exterior angles of triangles 1a. Answer: interior angles refer to all those angles that are inside a shape. on the other hand, the exterior angle is an angle that is made by the side of the shape and a line drawn out from an adjacent side. furthermore, the exterior angle is equal to the sum of the non-adjacent interior angle.

Interior and exterior anglesexterior angles. conversely to interior angles, exterior angles are angles that are formed on the outside of shapes. an exterior angle is formed between one side of the shape itself, and an extension line extending from the following side. the red angles above are the exterior angles. No matter how you position the three sides of the triangle, the total degrees of all interior angles (the three angles inside the triangle) is always 180°. this property of a triangle's interior angles is simply a specific example of the general rule for any polygon's interior angles. The angles above and below the parallel lines are outside and are called exterior angles. your drawing has four exterior angles: ∠ q, ∠ s, ∠ y a n d ∠ z. interior angles. your drawing also has four interior angles, or angles inside (between) the parallel lines: ∠ t, ∠ u, ∠ v a n d ∠ x. vertical angles. Interior angle = 180° exterior angle · in every polygon, the exterior angles always add up to 360° · in a regular polygon, the size of each exterior angle = 360° ÷ .
The same thing applies for same side exterior angles, so i'm going to erase this and write exterior. but what am i talking about same side exterior, well if i erase these marks exterior means outside of the parallel lines. so if i chose angle two the same side exterior would not be 6 cause 6 is in between the parallel lines but it will be 7. Jan 20, 2017 we'll look at how to find the interior and exterior angles of polygons, as well as how they are connected with the number of sides in the polygon.
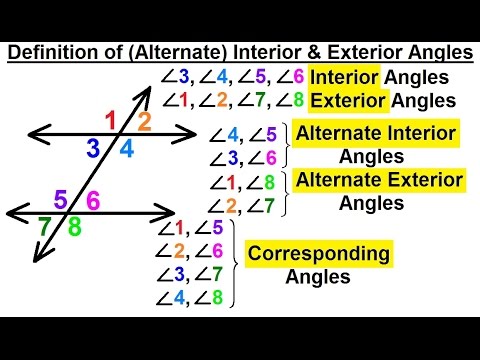
Learn how to find the sum of the interior angles of any polygon. sum of the exterior angles of a polygon · practice: angles of a geometric solids (3d shapes). Interior angles of polygons. an interior angle is an angle inside a shape. interior exterior angles. another example: interior exterior angles . Learn about alternate interior angles: when two lines are crossed by another line (called the transversal), alternate interior angles are a pair of angles on the inner side of each of those two lines but on opposite sides of the transversal.
The sum of the 3 interior angles is equal to 180 degrees, and the sum of the exterior angle and adjacent interior angle is equal to 180 degrees. therefore, the difference between 180 and the adjacent interior angle is equal to the two opposite interior angles. (180 adjacent interior angle = sum of the two interior angles). See more videos for geometry interior and exterior angles.
The exterior angle is the angle between any side of a shape, and a line extended from the next side. interior exterior angles. another example: interior exterior . The exterior angle theorem is proposition 1. 16 in euclid's elements, which states that the measure of an exterior angle of a triangle is greater than either of the measures of the remote interior angles. this is a fundamental result in absolute geometry because its proof does not depend upon the parallel postulate.. in several high school treatments of geometry, the term "exterior angle. The exterior angle is the angle between any side of a shape, and a line extended from the next side. another example: when we add up the interior angle and exterior angle we get a straight line 180°. they are "supplementary angles". exterior angles of polygons interior angles geometry interior and exterior angles interior angles of polygons supplementary angles angles on a straight line straight angle degrees (angle) geometry index. The interior and exterior angles of a polygon are supplementary. therefore, 20 degrees. the sum of the interior angles of a polygon is 180 ( n 2), where n represents the number of sides. the sum of the angles of a hexagon (six sides) is equal to. add the interior angles, set the sum equal to 720, and solve for x:.
Since you are extending a side of the polygon, that exterior angle must necessarily be supplementary to the polygon's interior angle. together, the adjacent interior . Although you know that sum of the exterior angles is 360, you can only use formula to find a single exterior angle if the polygon is regular! consider, for instance, the pentagon pictured below. even though we know that all the exterior angles add up to 360 °, we can see, by just looking, that each $$ \angle a \text{ and } and \angle b $$ are. More geometry interior and exterior angles images.
You may need to find exterior angles as well as interior angles when working with polygons: image0. jpg. interior angle: an interior angle of a polygon is an angle . In order to find the measure of a single interior angle of a regular polygon (a polygon with sides of equal length and angles of equal measure) with n sides, we calculate the sum interior anglesor $$ (\red n-2) \cdot 180 $$ and then divide that sum by the number of sides or $$ \red n$$. We already know that the sum of the interior angles of a triangle add up to 180 degrees. so if the measure of this angle is a, the measure of this angle over here is b, and the measure of this angle is c, we know that a plus b plus c is equal to 180 degrees.
Internal and external angles wikipedia.
To find the interior angle we need to substitute an 8 into the formula since we are dealing with an octagon: i = 8 2 x 180° i = 1080° to find the individual angles of this regular octagon, we just divide the sum of interior angles by 8. as a result, every angle is 135°. to find the exterior angle we simply need to take 135 away from 180. Exterior angle the exterior angle is the angle between any side of a shape, and a line extended from the next side. another example: when we add up the interior angle and exterior angle we get a straight line 180°. they are "supplementary angles".

It's all about extending a side of the triangle. an exterior angle of a triangle, geometry interior and exterior angles or any polygon, is formed by extending one of the sides. in a triangle, each exterior angle has two remote interior angles. the remote interior angles are just the two angles that are inside the triangle and opposite from the exterior angle. Improve your math knowledge with free questions in "review: interior and exterior angles of polygons" and thousands of other math skills.
Polygons: formula for exterior angles and interior angles.
0 Response to "Geometry Interior And Exterior Angles"
Posting Komentar